Solar Physics Historical Timeline (1223 BC - 200 BC)
Timeline
In this page
1223 BC: The oldest eclipse record
The oldest eclipse record is found on a clay tablet uncovered in the ancient city of Ugarit, in what is now Syria, with two plausible dates usually cited: 3 May 1375 BC or 5 March 1223 BC, the latter being favored by most recents authors on the topic.

Ancient ruins at Ugarit city, now Syria, where clay tablet with oldest eclipse record was discovered.
It is certainly clear that by the eight century BC, the Babylonians were keeping a systematic record of solar eclipses, and may even have been able to predict them fairly accurately based on numerological rules. Total eclipses of the Sun are arguably the most impressive astronomical phenomenon that can be observed more or less regularly with the naked eye (see slide 9 and slide 10 of the HAO slide set The Sun: A Pictorial Introduction). They occur when the Moon reaches a point in its orbit around the Earth that lies on the line joining the Earth and Sun. By a remarkable coincidence, the Moon's angular diameter, as seen from the Earth, is almost identical to that of the Sun. The Sun's disk is then completely eclipsed, and daytime darkness falls upon the Earth for a few minutes (this physical explanation of the phenomenon was only put forth much later, in the first century BC). Like comets, solar eclipses were taken to be astrological omens of great significance. It is therefore not surprising that such a spectacular event is often mentioned in surviving written records and chronicles of ancient civilizations.
References and further reading
- Fotheringham, J.K. 1932, The Story of Hi and Ho, Journal of the British Astronomical Association, 43, 248-257.
- Zirker, J.B. 1995, Total Eclipses of the Sun, Princeton University Press.
- Littman, M., Willcox, F., and Espenak, F. 2000, Totality: Eclipses of the Sun, 2nd ed., Oxford University Press.
ca. 800 BC: The first plausible recorded sunspot observation
The two oldest record of a sunspot observation are found in the Book of Changes, probably the oldest surviving Chinese book, compiled in China around, or before, 800 BC.
The text reads "A dou is seen in the Sun", and "A mei is seen in the Sun". From the context, the words (i.e., Chinese characters) "dou" and "mei" are taken to mean darkening or obscuration. Astronomers at the court of the Chinese and Korean emperors made regular notes of sunspots. It seems, however, that observations were not carried out systematically for their own sake, but instead took place whenever astrological prognostication was demanded by the emperor. The surviving sunspots records, though patchy and incomplete, cover nearly 2000 years and represents, by far, the most extensive pre-telescopic sunspot record.
Sunspots are concentrations of strong magnetic fields piercing the solar photosphere. Visually, they look like dark blemishes on the solar disk (see slide 1 and slide 3 of the HAO slide set). Most sunspots are too small to be readily visible by naked eye observations, but some reach a size sufficient to be visible without a telescope, under suitable viewing conditions (for example, when the sun is partially obscured by fog or thick mist, or clouds). Because of their possible astrological significance, reports of naked-eye sunspot observations are found in many ancient chronicles and court chronologies.
References and further reading
- Mossman, J.E., 1989, A comprehensive search for sunspots without the aid of a telescope, 1981-1982, in Quarterly J. R. Astr. Soc., 30, 59–73.
- Stephenson, F.R. 1990, Historical evidence concerning the Sun: interpretation of sunspot records during the telescopic and pre-telescopic eras, in Phil. Trans. R. Soc. London, A330, 499-512.
- Hetherington, B. 1996, A chronicle of pre-telescopic astronomy, John Wiley and Sons.
ca. 350 BC: Sun circling under a sheltering sky

The Aristotelian cosmos as modeled by Ptolemy. The Earth sits motionless at the center of the universe, and the outer sphere, the Primum Mobile, is assumed to undergo a full revolution in 24 hours.
One of the major intellectual achievements of ancient Greece is the physical model of the cosmos developed by Aristotle (384-322). An essential feature is the place occupied by the Earth at the center of the Universe, with the Sun, planets and sphere of fixed stars revolving about that center, the Sun occupying the fourth sphere. In this geocentric model the Earth is absolutely fixed, with the motions of procession and daily rotation ascribed to the two outermost spheres of the model.
This basic planetary arrangement formed the basis of a mathematical model of planetary motion developed four centuries later by Claudius Ptolemy (ca. 100-170). In Aristotle's scheme there existed fundamental physical differences between the terrestrial and celestial realms, as demarcated by the Moon's sphere. Everything inside the Moon is made of the four elements earth, water, air and fire, themselves arranged concentrically about the center of the universe. Pure circular motion prevails throughout the heavens, which are are made of an incorruptible fifth element (or "quintessence").
References and further reading
- Grant, E. 1977, Physical Science in the Middle Ages, Cambridge University Press
- Crowe, M.J. 1990, Theories of the World from Antiquity to the Copernican Revolution, Dover.
- Pedersen, O. 1993, Early Physics and Astronomy, revised ed., Cambridge University Press.
ca. 200 BC: The distance to the Sun
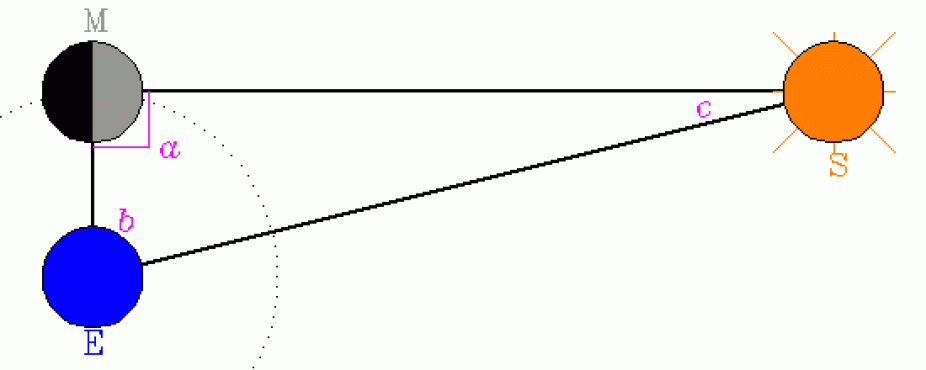
Aristarchus' geometric construction used to estimate the distance to the Sun. The Earth-Sun-Moon triangle and sizes are not drawn to scale.
The first mathematic-based attempt at determining the Sun-Earth distance is due to Aristarchus of Samos (ca. 310-230 BC). The procedure followed by Aristarchus is illustrated in this diagram: form a triangle by connecting the Earth (E), Sun (S) and Moon (M). At the first or third Moon quarter, the triangle so described is a right-angle triangle (a=90°). The angle b can be measured by an observer on Earth, which then allows the angle c to be computed (c=90-b when a=90°). The ratio of the Earth-Moon segment (EM) to the Earth-Sun segment (ES) is by definition equal to sin(c) (in modern trigonometric parlance -- Aristarchus expressed this differently).
While sound in theory, in practice this procedure is highly inaccurate in the Earth/Sun/Moon case; this is because EM is much smaller than ES, implying that b is very close to 90°, so that c is in turn very small. This has the consequence that a small measurement error on b translates in a large variation in the ratio EM/ES (again in modern parlance, a measurement error db is amplified by a factor 1/(sin c)2, which is large when c is very small). Aristarchus measured b=87°, while the true value is in fact 89° 50'. This may seem like a small error, but because of the large error amplification, Aristarchus' value leads to EM/ES=19, instead of the true value EM/ES=397. Nonetheless, Aristarchus' calculation was the first to mathematically set the spatial scale of the cosmos.
References and further reading
- Van Helden, A. 1985, Measuring the Universe, University of Chicago Press.
- Hirschfeld, A.W. 2001, Parallax, Freeman.